The lifetime of an unstable particle determines with nearly absolute certainty under which force it decays:
These are the accepted typical reaction times for W (weak force mediated by the weak particles W+ W- and Z0), P (Electromagnetic force mediated by Photons) and G (Strong force mediated by Gluons).
In paragraph 4 of page 2 of THE EXPANSION OF THE UNIVERSE is introduced that the photon consists of an electron and a positron coinciding massless: within the time border Higgs field absorption from the electron is canceled by Higgs field emission of the positron. The e- and e+ in the photon don't merge, they each keep all their separate properties.
In page 5 of NET FORCE IN QED is argued, when an electron in the atom emits a photon, the positron-electron pair needed to form this photon, originates from the shield of virtual particles surrounding the infinite strong electric core of the electron. It is concluded spin 0 photons should exist and they are dumped in the vacuum as the leptonic Higgs field, named so because I first thought the leptons absorb their mass from it.
In paragraph Four quarks in the shell at page 5 of QCD, is shown how pairs of quarks, taken out of the shell of virtual particles that surrounds each quark, can form colored spin 0 gluons. Here two quarks do merge, they couple and form a new gluon particle.
The three generations
At page 3 of NEWTON EINSTEIN GRAVITATION is argued Coupling to standing-still field, absorbing from standing-still field, takes least energy and is most abundant.
Mass should be absorbed from the field that is standing still with respect to the mass-absorbing particle because it leads to the lowest energy absorption. We pursue this argument here and state that the Higgs field from which mass is absorbed is the field of lowest energy.
Assumed is that the stronger the field, the faster it reacts and the more energy it contains. Therefore is assumed the colored gluon field contains most energy, the spin 0 photon field contains lesser energy and the neutrinophoton field contains least energy.
Then all particles of the 1rst generation (quarks u and d, the electron and positron, and the electron neutrinos) absorb their mass from the lowest energy field, the Higgs field that consists of neutrinophotons. For the stable particles we observe in the world around us, this suits better with the Standard Model as it is now: a Higgs field virtually without any properties and only meant for giving mass. We call the neutrinophoton Higgs field the 1rst Higgs field.
The particles of the 2nd generation (quarks s and c, the muon and the muon neutrinos) absorb their mass from what I called so far the leptonic Higgs field, or photonic Higgs field, made of spin 0 photons. Let's call it the 2nd Higgs field.
And finally the 3rd generation (quarks b and t, the tauon and tau neutrinos) should absorb their mass from the most energetic field, the gluon field made of spin 0 pairs of colored gluons. That field I called so far the hadronic Higgs field or gluonic Higgs field, and now best can be called the 3rd Higgs field *). This would only occur when there is plenty energy available for the particles to afford the higher mass absorption.
*) The 1rst generation absorbs mass from the 1rst Higgs field, the 2nd generation from the 2nd Higgs field, the 3rd generation from the 3rd Higgs field.
Why is not always absorbed from the lowest energy Higgs field? The reason might be the reaction time, making the colored reaction always faster and thus earlier occuring than the colorless color reaction, provided the energy needed for that is available. The 10^-23 sec reaction time of colored particles absorption from the vacuum is significant higher than the reaction time of colorless colors with electric charge - that is what we assume. While the reaction time of colorless colors with electric charge, like the electron and the positron, should be on its turn significantly higher than the reaction time of colorless colors without electric charge.
So a particle first tries to absorb mass from the fastest field, the hadronic Higgs field, if it can afford the energy. If not, then it tries to absorb from leptonic Higgs field, provided it can afford that. If it still hasn't enough energy for that, it finally absorbs from the lowest-energy field, the neutrinophoton-field. That is the ground state nearly all matter we know is in.
Energy must be an internal state. **) E.g. the quark composition uds, with spin 3/2, called the Σ*0 (sigma star null) particle. The u and d are in groundstate while the s is supposed to be in an excited state, e.g. circling around an u-d axis. Then, when it de-excitates and falls back to a lower energy state, it decays into e.g. Δ0 (delta null) = udd with spin 3/2.
**) It can't be the kinetic energy relative to e.g. the surrounding air molecules because air molecules are too absent in the particle accelerator machines. The particle track often is too short to entirely cross a single air molecule. The particle tracks then should only appear when accidentally within an air molecule.
The picture should not surprise. The main difference between the three generations is the mass of the particles. The Higgs field gives mass, that's the only thing it does. So one Higgs field for each generation is an obvious possibility.
Bose-Einstein condensates
The vacuums made of vacuum particles must be Bose condensates.
In State 4 at page 5 of NEG is said: The gravitational field in action is supposed to be a liquid Bose condensate.
Propagation speed at page 6 of NEG says The vacuum is thought of as a bose condensate, ruled by one single wave function. The sagging‑in shell after shell doesn’t very much look like one single wave function. It rather is a sequence of distinct processes. So for the vacuum to remain a bose condensate maybe the entire vacuum react as a whole, all sagging‑ins in one single stroke (all sagging‑ins that are the result of one marble absorption, one hole), in the frame of reference wherein the vacuum marble was standing still. Subsequent holes filled in by new sagging-ins, those then are subsequent distinct processes.
Why refer to Bose condensates again? In formulating the three generations as just have been done, there seems to rise a problem. The 3rd Higgs field, made of gluon pairs of spin 1, can in principle be considered to form a grid: the colored gluons of that vacuum cohere, in doing so giving the vacuum its coherence. But photons as well as neutrinophotons don't cohere, I can't imagine how they would. Spin 0 photons and spin 0 neutrinophotons do form the 2nd and 1rst Higgs field respectively but they don't form a grid. Then the Higgs mechanism of absorbing a Higgs vacuum particle will work, but the subsequent act of gravitation, the subsequent streaming of the vacuum particles into the hole (the liquid state), will not. The restoring of the grid after the streaming, the return to solid state vacuum, is of no application.
So the entire 1rst and 2nd generation would not gravitate. From photons is derived they don't gravitate: the constituting e+ and e- cancel each others Higgs field absorption/emission. For the electrons and fellow neutrinos one eventually could start considering that they don't gravitate either. But that the quarks u, d, s and c wouldn't gravitate - the gravitation has to come from somewhere!
So to maintain the view build up in this website so far, I am forced to state that the spin 0 vacuum photons as well as the spin 0 vacuum neutrinophotons do form a grid. And the only way I see how they can do that is to assume they are Bose condensates.
Would that do? It would answer the question about the propagation speed of the sagging-in of shell after shell of vacuum particlesin paragraph Propagation speed
at page 6 of NEG (storyline NEWTON EINSTEIN GRAVITATION).
Moreover, spin 0 photons nor spin 0 neutrinophotons will ever add to the spin 2 of the graviton. Spin 1 photons can align to a spin 2 graviton vacuum particle, but two photons do not cohere just like that. Spin 1 neutrinophotons even do not exist. So I conclude The spin consideration at page 3 of QG, especially conclusion (3.6), is a 3rd generation feature only.
Everything can couple to everything by color force. Examining the Standard Model.
In the course of this website is found that all particles of the standard model have color, although a number of them have the colorless color +1 or -1 (quaternion +1 or -1). The gluon consists of two colored quarks, massless coinciding within their timeborders. The photon consists likewise of an electron and a positron massless coinciding, the e+ and e- being quarks with color +1 and -1 and electric charge. The neutrinos are quarks with color +1 or -1 without electric charge. We always take particles to have color (quaternion value +1, i, j, k) and antiparticles to have anticolor (quaternion value -1, -i, -j, -k).
Regard paragraph Four quarks in the shell
at page 5 of QCD, the paragraph starting with Mark the = pairs in the 3rd and 4th scheme are interesting, nearly at the end of the paragraph. If we indeed take this to be the W+ and W- particle, we in fact have identified all particles from the Standard Model with quarks, particles that somehow feel the strong force G. Except for the Z0, I still didn't found that.
In Four quarks in the shell
W+ has quark composition u d and W- has quark composition u d. (d means anti d quark
, etc.) Our supposed W+ and W- have the freedom to have any of the colors 1, i, j, k, -1, -i, -j, -k. The particles with color i, j, k, -i, -j, -k glue. Let's take the freedom to identify this with the 
meson with mass 140 MeV. Our supposed W+ and W- with colorless color +1 or -1 don't glue but only couple by G; this is very comparable with the W+ and W- from the standard model that only couple but do not mediate a force. Let's take this as the true W from the standard model with mass 80385 MeV.
So everything couples to everything else by G (strong nuclear force), although not every coupling glues. Regard the scheme of the standard model below. How do the non-existing grey lines in the standard model work out? I can't calculate but I can make an estimation. The difference with the standard model is the supposed coupling by G, the rest is the same as in the standard model.
Mark we don't try to identify force fields with each other, see page 7, paragraph Can electric charge be identified with colorless quaternion color?. Electric charge is quite something else than color charge.
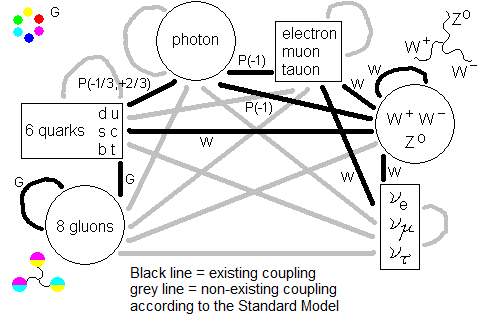
Let's call a non-existing coupling (according to the standard model) by the two particles the grey line connencts, separated by a small piece of grey line 
, gl = gluon, q = quark, ph = photon, e = electron or positron. We don't immediately bother which particle absorbs which. At the moment they couple, the particles merge to a merger particle and that's all there is for the moment.
The gluon has color [ i, j, k, -1, -j, -k ] and only will be found in the very neighborhood of quarks.
OR the gluon has the colorless color
+1 or -1 and is a glueball, see the free mesonic gluon in fig 3.9 at page 3 of QCD.
Here [ i, j, k, -i, -j, -k ] means one element out of the set [ i, j, k, -i, -j, -k ]
, etc. So [ i, j, k ] means i, or it means j, or it means k.
When both merging particles have mass the mass of the merger line should not be taken as it is given here. The result is given between ( ).
Color 
e = +1, color 
e = -1, see color of the neutrino at the previous page.
Mass merger = gl mass + mass = 0 + mass = mass
spin merger = spin gl + spin = -1 +1/2 () = -1/2. Or spin merger = +1 -1/2 () = +1/2
Electric charge merger = 0 + 0 = 0
Color merger = color gl * color = [ 1, i, j, k, -1, -i, -j, -k ] * [ -1, +1 ] = [ 1, i, j, k, -1, -i, -j, -k ].
When color = +1, then the merger is a particle with the same color or anticolor as the gluon it couples with.
When color = -1, then the merger is a particle with the opposite color or anticolor of the gluon it couples with.
When the color of the gluon was [ i, j, k, -i, -j, -k ] then the merger cannot leave the confinement the gluon was in. Moreover, the gluon was part of a process of color interchange, there is no other place for colored gluons, and that gluon now is missing. I suppose the merger particle cannot take over the color task of the missing gluon for several reasons (mass, spin). I am not sure what happens then, but I guess the merger splits again in the original gluon and neutrino.
When the color of the gluon was +1 then the merger is a particle with neutrino mass, spin [ -1/2, +1/2], zero electric charge and same color as the neutrino it coupled with. So the merger is a neutrino. However, it are spin -1/2 and spin +1/2 which do not occur. This reaction will not take place.
When the color of the gluon was -1 then the merger is a particle with neutrino mass, spin [ -1/2, +1/2], zero electric charge and opposite color as the neutrino it coupled with. This means the has become an , or the has become a . The coupling with the gluon changed the spin into its opposite too. This results in existing spin +1/2 and spin -1/2 . So I conclude, when the color -1 gluon is not part of a color interchange process in a hadron, in other words when the color -1 gluon has escaped into outer space, then the color -1 gluon indeed couples with a neutrino to yield the opposing existing neutrino.
gl W+ W- Z0
Mass merger = mass gl + mass [ W+, W-, Z0 ] = 0 + mass [ W+, W-, Z0 ] = mass [ W+ W- Z0 ]
Spin merger = spin gl + spin [ W+ W- Z0 ] = 1 + 1 = 2, or -1 -1 = -2, or 1 - 1 = 0.
Electric charge merger = 0 + [ -1, +1 ] = [ -1, +1 ]
Color merger = color gl * color [ W+ W- Z0 ] = [ 1, i, j, k, -1, -i, -j, -k ] * 0 = 0
The merger particle has mass W+ W- or mass Z0, spin -2, 0 or +2, electric charge +1 or -1 and no color (0 is not a color). Color W = 0, see The color of W at the previous page. So the gluon and the W+, W- and Z0 don't react with the gluon by G.
gl e, mu, tau
The color of e- is -1, the color of e+ is +1, see paragraph Electrons as Baryons.
Mass merger = mass gl + mass [ e, mu, tau ] = 0 + mass [ e, mu, tau ] = mass [ e, mu, tau ].
Spin merger = spin gl + spin [ e, mu, tau ] = 1 - 1/2 = +1/2. Or spin merger = -1 +1/2 = -1/2.
Electric charge merger = 0 +1 = +1 or 0 -1 = -1
Color merger = color gl * color [ e, mu, tau ] = [ 1, i, j, k, -1, -i, -j, -k ] * [ +1, -1 ] = [ 1, i, j, k, -1, -i, -j, -k ]
The merger is a particle of mass [ e, mu, tau ], spin [ -1/2, +1/2], electric charge [ +1, -1 ] and color [ +1, -1 ].
The interaction between the color of e+ e- and the color of the quarks is discussed in paragraph Electrons as Baryons at the previous page. The same reasoning holds for the interaction between e+ e- and the gluons in the hadrons.
Coupling e+ e- with a gluon of color +1 (somewhere in free space) leads to spin swap of the e+ e-, which is a permitted state change.
Coupling e+ with gluon of color -1, would that change it into e-? Which violates electric charge conservation. Or only give a positron with an anticolor? Which will not be observable, but is not was is agreed upon. Ditto for coupling e- with color -1 gluon. I conclude the reaction will not take place. Ditto for mu and tau particles.
gl ph
Mass merger = gl mass + ph mass = 0 + 0 = 0
Spin merger = spin gl + spin ph = 1 + 1 = 2. Or spin merger = 1 - 1 = 0.
Electric charge merger = 0 + 0 = 0
Color merger = color gl * color ph = [ 1, i, j, k, -1, -i, -j, -k ] * 0 = 0
The color of the photon is zero and the color of the merger is zero. Zero is not a quaternion unit, is not a color. So I conclude ph and gl don't couple by G.
Color e = +1, color e = -1, see color of the neutrino at the previous page.
Mass merger = (mass + mass quark)
Spin merger = 1/2 + 1/2 = 1. Or 1/2 - 1/2 = 0.
Electric charge merger = 0 +2/3 = +2/3. Or 0 -1/3 = -1/3.
Color merger = [ +1, -1 ] * [ i, j, k, -i, -j, -k ] = [ i, j, k, -i, -j, -k ]
The merger has more or less one quark mass, spin [ -1, 0, 1 ], electric charge [ -1/3, +2/3 ] and color [ i, j, k, -i, -j, -k ].
In the standard model the neutrino is supposed not to react with a quark directly. The neutrino couples with W+ W- Z0 and W+ W- Z0 couples with a quark, that's the rule. If every particle has a kind of color, as is suggested in this website, then in principle there is a contribution of neutrino's directly coupling to quarks.
The e with its color -1 cannot be absorbed by a quark in a baryon or meson because it would leave the baryon or meson colored, compare 1) in Electrons as Baryons
at previous page. So the e is not observed by the baryon or meson, they doesn't see the e.
The spin +1/2 e has color 1. This, as far as color is concerned, makes the e extremely vulnerable for absorption by any quark via the strong nuclear force, compare 2) in Electrons as baryons
. But then again, when spin merger is 1, where to leave the extra spin +1/2? There is no other way than emit an e again. When spin merger is zero I guess the merger has a need for a spin 1/2, and where to get that? So yes, the e reacts extremely easily with the quarks in the baryon or meson, but no, they don't stay there. This does hold as an observation, the e is seen by the baryon or meson, but it never causes a state change.
Well, that is, two simultaneous neutrinos e and e can do the job: within one and the same 10^-23 sec two e happen to coincide near a quark in a proton or neutron. The two neutrinos then merge by the strong nuclear force to a spin -1 particle of color -1 x -1 = 1 that is immediately absorbed by the quark. The quark then emits the extra spin 1 by one photon. It seems possible, but doesn't sound as an easy process.
Color e = +1, color e = -1, see color of the neutrino at the previous page.
Mass merger = mass + mass ph = mass + 0 = mass
Spin merger = [ -1/2, +1/2 ] + [ -1, +1 ] = [ -1/2, +1/2 ]
Electric charge merger = 0 + 0 = 0
Color merger = [ color ] * [ color ph ] = [ -1, +1 ] * 0 = 0
The merger is a particle with mass, spin [ -1/2, +1/2 ], no electric charge and color 0.
The color of the photon is zero and the color of the merger is zero. Zero is not a quaternion unit, is not a color. So I conclude ph and don't couple by G.
u, d, s, c, b, t e, mu, tau
Mass merger = (mass [ u, d, s, c, b, t ] + mass [ e, mu, tau ] )
Spin merger = [ 1/2, -1/2 ] + [ 1/2, -1/2 ] = [ -1 , 0, 1 ]
Electric charge merger = [ +2/3, -1/3 ] + [ 1, -1 ] = [ +5/3, +2/3, -1/3, -4/3 ]
Color merger = [ i, j, k ] * [ +1, -1 ] = [ i, j, k, -i, -j, -k ]
The merger particle has unknown mass, spin [ -1 , 0, 1 ], electric charge [ +5/3, +2/3, -1/3, -4/3 ] and color [ i, j, k, -i, -j, -k ].
The interaction between the color of e+ e- and the color of the quarks is discussed in paragraph Electrons as Baryons. u, d, s, c, b, t in the baryon or meson always have color [ i, j, k, -i, -j, -k ]. Coupling e+ e- with a quark of color +1 somewhere in free space - such a quark, isn't that just the positron? After ECR? (Electric charge redistribution, obviously necessary to yield an observable particle.)
A spin 1 particle is not a quark, nor an electron. There has to be emitted a spin 1/2.
I conclude the reaction will not take place. Ditto for mu and tau particles.
u, d, s, c, b, t u, d, s, c, b, t
Mass merger = (mass [ u, d, s, c, b, t ] + mass [ u, d, s, c, b, t ] )
Spin merger = [ 1/2, -1/2 ] + [ 1/2, -1/2 ] = [ -1 , 0, 1 ]
Electric charge merger = [ +2/3, -1/3 ] + [ +2/3, -1/3 ] = [ -2/3, +1/3, +4/3 ]
Color merger = [ i, j, k ] * [ i, j, k ] = [ i, j, k, -1, -i, -j, -k ]
The merger particle has unknown mass, spin [ -1 , 0, 1 ], electric charge [ -4/3, -1/3, +1/3, +4/3 ] and color [ i, j, k, -1, -i, -j, -k ]. The color +1 seems to be lacking.
This reaction is already discussed in the standard model. The standard model says that quarks don't couple by G. Quarks and gluons couple, but quarks directly to quarks do not. Gluons couple with each other by G, so why wouldn't quarks do likewise?
A spin 1 particle is not a quark, a spin 1/2 has to be removed by e.g. a neutrino.
We take for granted that the reaction will not take place.
ph ph
Mass merger = mass ph + mass ph = 0 + 0 = 0
Spin merger = [ +1, -1 ] + [ +1, -1 ] = [ -2, 0, +2 ]
Electric charge merger = 0 + 0 = 0
Color merger = 0 + 0 = 0
The merger particle has no mass, spin [ -2, 0, +2 ], no electric charge and no color.
The color of the photon is zero and the color of the merger is zero. Zero is not a quaternion unit, is not a color. So I conclude ph and ph don't couple by G.
e, mu, tau e, mu, tau
The color of e- is -1, the color of e+ is +1, see paragraph Electrons as Baryons.
Mass merger = ( mass [ e, mu, tau ] + mass [ e, mu, tau ] )
Spin merger = [ -1/2, +1/2 ] + [ -1/2, +1/2 ] = [ -1 , 0, 1 ]
Electric charge merger = [ +1, -1 ] + [ +1, -1 ] = [ -2, 0, +2 ]
Color merger = [ -1, +1 ] * [ -1, +1 ] = [ -1, +1 ]
The merger particle has unknown mass, spin [ -1 , 0, 1 ], electric charge [ -2, 0, +2 ] and color [ -1, +1 ].
When e+ and e- coincide massless they form the photon, but then they don't merge.
Could e+ and e- merge? The electric charge -2 and +2 is an integer charge, so not immediately forbidden. However, we find it only at the Δ++ particle with quark composition uuu. Let's suppose it somehow is forbidden. So electric charge 0 is left. Then it only can be the pairs e+ e-, mu+ mu- and tau+ tau- that can merge. The color then always yield +1 * -1 = -1, the dangerous colorless color. The merger will not be absorbed by a p+ or n0 since it would leave them colored which is forbidden. We now have a particle with some mass, spin -1, 0 or +1, no electric charge and color -1. Do we recognize Z0? Did we finally found Z0, our last missing particle?
Color e = +1, color e = -1, see color of the neutrino at the previous page.
Mass merger = ( mass + mass )
Spin merger = [ -1/2, +1/2 ] + [ -1/2, +1/2 ] = [ -1 , 0, 1 ]
Electric charge merger = 0 + 0 = 0
Color merger = [ (color spin +1/2 e), (color spin -1/2 e) ] * [ (color spin +1/2 e), (color spin -1/2 e) ] = [ -1, +1 ] * [ -1, +1 ] = [ -1, +1 ].
Neutrinos don't glue but couple to each other by G, that's the consequence of the view so far. The merger particle has unknown (but maybe small) mass, spin [ -1 , 0, 1 ], no electric charge and color [ -1, +1 ].
A spin 1 particle is not a neutrino, a spin 1/2 has to be removed by... a neutrino. So after all the number of neutrinos doesn't change.
Final conclusion
The provisional final conclusion is that there is no objecting reaction/coupling, and thus no objection, to the view of every elementary particle of the Standard Model having a color.
Some final remarks
- The spin 0 photon bosecondensate and the spin 0 neutrinophoton bosecondensate maybe are too only short-living intermediate states between THE spin 2 graviton field and the Higgs field absorbing matter particle , just like described in paragraph The Spin Consideration at page 3 of QG. But how? Spin 0 photons cannot form spin 2 gravitons.